At Rowley Park Academy, we want children to be competent in maths for the rest of their lives: not just in the short-term. We strive to do this by following the three maths curriculum aims, they are:
Fluency
Children become fluent in the fundamentals of mathematics, including through varied and frequent practice with increasingly complex problems over time, so that pupils develop conceptual understanding and the ability to recall and apply knowledge rapidly and accurately.
Reasoning
Children reason mathematically by following a line of enquiry, conjecturing relationships and generalisations, and developing an argument, justification or proof using mathematical language
Problem solving
Children solve problems by applying their mathematics to a variety of routine and non-routine problems with increasing sophistication, including breaking down problems into a series of simpler steps and persevering in seeking solutions.
How do we teach maths at Rowley Park Academy?
Maths is an interconnected subject in which pupils need to be able to move fluently between representations of mathematical ideas. Pupils’ understanding and their readiness to progress is at the forefront of every teacher’s mind before they are moved to the next stage. For pupils who grasps concepts rapidly, they are challenged through a range of contexts, being offered rich and sophisticated problems before acceleration through new content.
Examples of each curriculum aim and how we teach them in our maths lessons are detailed below:
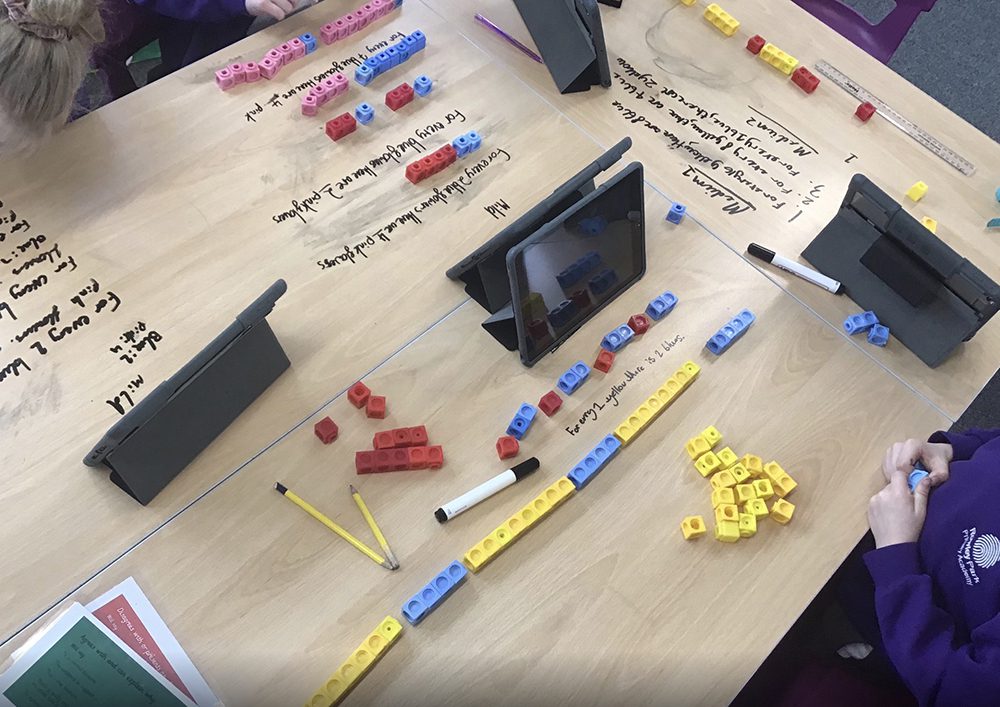
Fluency
We encourage fluency by teaching via a CPA approach (Concrete, Pictorial and Abstract) where children have the opportunity to manipulate mathematical objects such as place value counters and Numicon so that they have a better conceptual understanding before moving onto the pictorial stage and finally the advanced stage, the abstract stage. To support the teaching of fluency, daily arithmetic sessions are delivered to ensure children are offered a varied and frequent practice.

Reasoning
Every maths lesson at RPA is focused on an element of reasoning. Classroom staff question and probe children’s choices of methods and mathematical vocabulary so that every lesson encourages a debate around which is the correct or most efficient method. This is supported by our use of thinking frames, which encourage children to think about their mathematical thinking, which both develop their mathematical reasoning and decision-making.
Problem Solving
Like the above aims, problem solving is intertwined within the daily lesson so that children have the confidence to solve problems which include different contexts. Our big pictures encourage children to think with sophistication, breaking down problems with the use of strategies such as bar modelling and persevering through the use of making links across the whole maths curriculum.

Daily maths lessons consist of:
- Arithmetic starter to learn, practice and reinforce times tables, number and the four operations.
- Whole class teaching and modelling of a concept.
- Arithmetic and problem solving activities, differentiated to meet the needs of all children.
- Extension activities to promote mastery maths and deeper understanding.
- Plenaries to consolidate, challenge and extend through arithmetic, reasoning and problem solving.
Parents
To become fluent and confident in their understanding of maths, children need practise to acquire a deep, long-term and secure understanding. We ask all parents to practise place value, the four operations (addition, subtraction, multiplication and division) and times tables as much as possible with their children.
By the end of KS1, children should be fluent in their 2, 3, 4, 5 and 10 times tables
By the end of Year 4, children should be fluent in all their times tables up to 12 x 12
This fluency will allow them to access their learning in lessons with more confidence. Maths will be supported at home through Times Table Rockstars and MyMaths.
Curriculum Maps for Progress in Understanding Mathematics Assessment
The PUMA tests provide thorough coverage of the new National Curriculum Programme of Study for the particular year. These Curriculum Maps take in the new Programme of Study, which describes what should be covered by the end of each year, and suggest how teaching of the material might be allocated to each term.